Multispheroidal model of magnetic field of uncertain extended energy-saturated technical object
DOI:
https://doi.org/10.20998/2074-272X.2025.1.07Keywords:
energy-saturated extended technical objects, magnetic field, multispheroidal model, magnetic silencing, extended spheroidal coordinate system, spatial extended spheroidal harmonicsAbstract
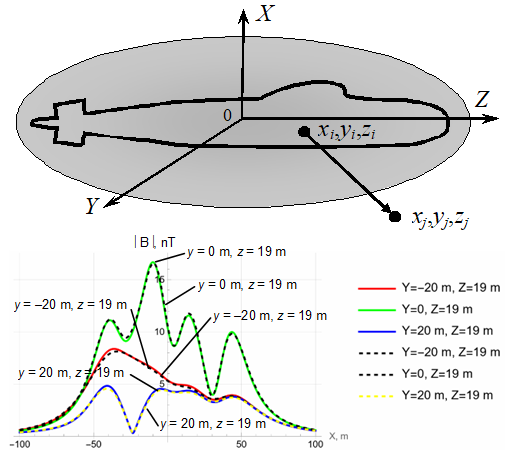
Problem. The implementation of strict requirements for magnetic silence of elongated energy-saturated technical objects – such as naval vessel and submarines is largely determined by the adequacy of mathematical models to the signatures of a real magnetic field. Aim. Simplification of mathematical modeling of the magnetic field of an uncertain extended energy-saturated object based on the development and application of a multispheroidal model of its magnetic field instead of the well-known multidipole model. Methodology. Coordinates of the geometric location and magnitudes of spatial extended spheroidal harmonics of spheroidal sources of multispheroidal model of magnetic field calculated as magnetostatics geometric inverse problems solution in the form of nonlinear minimax optimization problem based on near field measurements for prediction far extended technical objects magnetic field magnitude. Nonlinear objective function calculated as the weighted sum of squared residuals between the measured and predicted magnetic field COMSOL Multiphysics software package used. Nonlinear minimax optimization problems solutions calculated based on particle swarm nonlinear optimization algorithms. Results. Results of prediction far magnetic field magnitude of extended technical objects based on designed multispheroidal model of the magnetic field in the form of spatial prolate spheroidal harmonics in prolate spheroidal coordinate system using near field measurements with consideration of extended technical objects magnetic characteristics uncertainty. Originality. For the first time the method for design of multispheroidal model of magnetic field of uncertain extended energy-saturated technical object based on magnetostatics geometric inverse problems solution and magnetic field spatial spheroidal harmonics calculated in prolate spheroidal coordinate system taking into account of technical objects magnetic characteristics uncertainties developed. Practical value. It is shown the possibility to reduce the number of spheroidal sources of the magnetic field for adequate modeling of the real magnetic field based on the developed multispheroidal model compared to the number of well-known dipole sources of the magnetic field in the multidipole model of the magnetic field. References 48, figures 4.
References
Rozov V.Yu., Getman A.V., Petrov S.V., Erisov A.V., Melanchenko A.G., Khoroshilov V.S., Schmidt I.R Spacecraft magnetism. Technical Electrodynamics. Thematic issue «Problems of modern electrical engineering», 2010, part 2, pp. 144-147. (Rus).
Rozov V.Yu. Methods for reducing external magnetic fields of energy-saturated objects. Technical Electrodynamics, 2001, no. 1, pp. 16-20.
Rozov V.Yu. Selective compensation of spatial harmonics of the magnetic field of energy-saturated objects. Technical Electrodynamics, 2002, no. 1, pp. 8-13. (Rus).
ECSS-E-HB-20-07A. Space engineering: Electromagnetic compatibility hand-book. ESA-ESTEC. Requirements & Standards Division. Noordwijk, Netherlands, 2012. 228 p.
Droughts S.A., Fedorov O.P. Space project Ionosat-Micro. Monograph. Kyiv, Akademperiodika Publ., 2013. 218 p. (Rus).
Holmes J.J. Exploitation of A Ship’s Magnetic Field Signatures. Springer Cham, 2006. 67 p. doi: https://doi.org/10.1007/978-3-031-01693-6.
Woloszyn M., Jankowski P. Simulation of ship’s deperming process using Opera 3D. 2017 18th International Symposium on Electromagnetic Fields in Mechatronics, Electrical and Electronic Engineering (ISEF) Book of Abstracts, 2017, pp. 1-2. doi: https://doi.org/10.1109/ISEF.2017.8090680.
Birsan M., Holtham P., Carmen. Using global optimisation techniques to solve the inverse problem for the computation of the static magnetic signature of ships. Defense Research Establishment Atlantic, 9 Grove St., PO Box 1012, Dartmouth, Nova Scotia, B2Y 3Z7, Canada.
Zuo C., Ma M., Pan Y., Li M., Yan H., Wang J., Geng P., Ouyang J. Multi-objective optimization design method of naval vessels degaussing coils. Proceedings of SPIE - The International Society for Optical Engineering, 2022, vol. 12506, art. no. 125060J. doi: https://doi.org/10.1117/12.2662888.
Mehlem K., Wiegand A. Magnetostatic cleanliness of spacecraft. 2010 Asia-Pacific International Symposium on Electromagnetic Compatibility, 2010, pp. 936-944. doi: https://doi.org/10.1109/APEMC.2010.5475692.
Messidoro P., Braghin M., Grande M. Magnetic cleanliness verification approach on tethered satellite. 16th Space Simulation Conference: Confirming Spaceworthiness into the Next Millennium, 1991, pp. 415-434.
Mehlem K., Narvaez P. Magnetostatic cleanliness of the radioisotope thermoelectric generators (RTGs) of Cassini. 1999 IEEE International Symposium on Electromagnetic Compatability, 1999, vol. 2, pp. 899-904. doi: https://doi.org/10.1109/ISEMC.1999.810175.
Eichhorn W.L. Magnetic dipole moment determination by near-field analysis. Goddard Space Flight Center. Washington, D.C., National Aeronautics and Space Administration, 1972. NASA technical note, D 6685. 87 p.
Rozov V.Yu., Dobrodeev P.N., Volokhov S.A. Multipole model of a technical object and its magnetic center. Technical Electrodynamics, 2008, no. 2, pp. 3-8. (Rus).
Rozov V.Yu., Getman A.V., Kildishev A.V. Spatial harmonic analysis of the external magnetic field of extended objects in a prolate spheroidal coordinate system. Technical Electrodynamics, 1999, no. 1, pp. 7-11. (Rus).
Rozov V.Yu. Mathematical model of electrical equipment as a source of external magnetic field. Technical Electrodynamics, 1995, no. 2, pp. 3-7. (Rus).
Volokhov S.A., Dobrodeev P.N., Ivleva L.F. Spatial harmonic analysis of the external magnetic field of a technical object. Technical Electrodynamics, 1996, no. 2, pp. 3-8. (Rus).
Getman A.V. Analysis and synthesis of the magnetic field structure of technical objects on the basis of spatial harmonics. Dissertation thesis for the degree of Doctor of Technical Sciences. Kharkiv, 2018. 43 p. (Ukr).
Xiao C., Xiao C., Li G. Modeling the ship degaussing coil’s effect based on magnetization method. Communications in Computer and Information Science, 2012, vol. 289, pp. 62-69. doi: https://doi.org/10.1007/978-3-642-31968-6_8.
Wołoszyn M., Jankowski P. Ship’s de-perming process using coils lying on seabed. Metrology and Measurement Systems, 2019, vol. 26, no. 3, pp. 569-579. doi: https://doi.org/10.24425/mms.2019.129582.
Fan J., Zhao W., Liu S., Zhu Z. Summary of ship comprehensive degaussing. Journal of Physics: Conference Series, 2021, vol. 1827, no. 1, art. no. 012014. doi: https://doi.org/10.1088/1742-6596/1827/1/012014.
Getman A.V. Spatial harmonic analysis of the magnetic field of the sensor of the neutral plasma component. Eastern European Journal of Advanced Technologies, 2010, vol. 6, no. 5(48), pp. 35-38. doi: https://doi.org/10.15587/1729-4061.2010.3326.
Getman A. Ensuring the Magnetic Compatibility of Electronic Components of Small Spacecraft. 2022 IEEE 3rd KhPI Week on Advanced Technology (KhPIWeek), 2022, no. 1-4. doi: https://doi.org/10.1109/KhPIWeek57572.2022.9916339.
Acuña M.H. The design, construction and test of magnetically clean spacecraft – a practical guide. NASA/GSFC internal report. 2004.
Junge A., Marliani F. Prediction of DC magnetic fields for magnetic cleanliness on spacecraft. 2011 IEEE International Symposium on Electromagnetic Compatibility, 2011, pp. 834-839. doi: https://doi.org/10.1109/ISEMC.2011.6038424.
Lynn G.E., Hurt J.G., Harriger K.A. Magnetic control of satellite attitude. IEEE Transactions on Communication and Electronics, 1964, vol. 83, no. 74, pp. 570-575. doi: https://doi.org/10.1109/TCOME.1964.6539511.
Junge A., Trougnou L., Carrubba E. Measurement of Induced Equivalent Magnetic Dipole Moments for Spacecraft Units and Components. Proceedings ESA Workshop Aerospace EMC 2009 ESA WPP-299, 2009, vol. 4, no. 2, pp. 131-140.
Matsushima M., Tsunakawa H., Iijima Y., Nakazawa S., Matsuoka A., Ikegami S., Ishikawa T., Shibuya H., Shimizu H., Takahashi F. Magnetic Cleanliness Program Under Control of Electromagnetic Compatibility for the SELENE (Kaguya) Spacecraft. Space Science Reviews, 2010, vol. 154, no. 1-4, pp. 253-264. doi: https://doi.org/10.1007/s11214-010-9655-x.
Boghosian M., Narvaez P., Herman R. Magnetic testing, and modeling, simulation and analysis for space applications. 2013 IEEE International Symposium on Electromagnetic Compatibility, 2013, pp. 265-270. doi: https://doi.org/10.1109/ISEMC.2013.6670421.
Mehlem K. Multiple magnetic dipole modeling and field prediction of satellites. IEEE Transactions on Magnetics, 1978, vol. 14, no. 5, pp. 1064-1071. doi: https://doi.org/10.1109/TMAG.1978.1059983.
Thomsen P.L., Hansen F. Danish Ørsted Mission In-Orbit Experiences and Status of The Danish Small Satellite Programme. Annual AIAA/USU Conference on Small Satellites, 1999, pp. SSC99-I–8.
Kapsalis N.C., Kakarakis S.-D.J., Capsalis C.N. Prediction of multiple magnetic dipole model parameters from near field measurements employing stochastic algorithms. Progress In Electromagnetics Research Letters, 2012, vol. 34, pp. 111-122. doi: https://doi.org/10.2528/PIERL12030905.
Solomentsev O., Zaliskyi M., Averyanova Y., Ostroumov I., Kuzmenko N., Sushchenko O., Kuznetsov B., Nikitina T., Tserne E., Pavlikov V., Zhyla S., Dergachov K., Havrylenko O., Popov A., Volosyuk V., Ruzhentsev N., Shmatko O. Method of Optimal Threshold Calculation in Case of Radio Equipment Maintenance. Data Science and Security. Lecture Notes in Networks and Systems, 2022, vol. 462, pp. 69-79. doi: https://doi.org/10.1007/978-981-19-2211-4_6.
Ruzhentsev N., Zhyla S., Pavlikov V., Volosyuk V., Tserne E., Popov A., Shmatko O., Ostroumov I., Kuzmenko N., Dergachov K., Sushchenko O., Averyanova Y., Zaliskyi M., Solomentsev O., Havrylenko O., Kuznetsov B., Nikitina T. Radio-Heat Contrasts of UAVs and Their Weather Variability at 12 GHz, 20 GHz, 34 GHz, and 94 GHz Frequencies. ECTI Transactions on Electrical Engineering, Electronics, and Communications, 2022, vol. 20, no. 2, pp. 163-173. doi: https://doi.org/10.37936/ecti-eec.2022202.246878.
Havrylenko O., Dergachov K., Pavlikov V., Zhyla S., Shmatko O., Ruzhentsev N., Popov A., Volosyuk V., Tserne E., Zaliskyi M., Solomentsev O., Ostroumov I., Sushchenko O., Averyanova Y., Kuzmenko N., Nikitina T., Kuznetsov B. Decision Support System Based on the ELECTRE Method. Data Science and Security. Lecture Notes in Networks and Systems, 2022, vol. 462, pp. 295-304. doi: https://doi.org/10.1007/978-981-19-2211-4_26.
Shmatko O., Volosyuk V., Zhyla S., Pavlikov V., Ruzhentsev N., Tserne E., Popov A., Ostroumov I., Kuzmenko N., Dergachov K., Sushchenko O., Averyanova Y., Zaliskyi M., Solomentsev O., Havrylenko O., Kuznetsov B., Nikitina T. Synthesis of the optimal algorithm and structure of contactless optical device for estimating the parameters of statistically uneven surfaces. Radioelectronic and Computer Systems, 2021, no. 4, pp. 199-213. doi: https://doi.org/10.32620/reks.2021.4.16.
Volosyuk V., Zhyla S., Pavlikov V., Ruzhentsev N., Tserne E., Popov A., Shmatko O., Dergachov K., Havrylenko O., Ostroumov I., Kuzmenko N., Sushchenko O., Averyanova Yu., Zaliskyi M., Solomentsev O., Kuznetsov B., Nikitina T. Optimal Method for Polarization Selection of Stationary Objects Against the Background of the Earth’s Surface. International Journal of Electronics and Telecommunications, 2022, vol. 68, no. 1, pp. 83-89. doi: https://doi.org/10.24425/ijet.2022.139852.
Zhyla S., Volosyuk V., Pavlikov V., Ruzhentsev N., Tserne E., Popov A., Shmatko O., Havrylenko O., Kuzmenko N., Dergachov K., Averyanova Y., Sushchenko O., Zaliskyi M., Solomentsev O., Ostroumov I., Kuznetsov B., Nikitina T. Practical imaging algorithms in ultra-wideband radar systems using active aperture synthesis and stochastic probing signals. Radioelectronic and Computer Systems, 2023, no. 1, pp. 55-76. doi: https://doi.org/10.32620/reks.2023.1.05.
Maksymenko-Sheiko K.V., Sheiko T.I., Lisin D.O., Petrenko N.D. Mathematical and Computer Modeling of the Forms of Multi-Zone Fuel Elements with Plates. Journal of Mechanical Engineering, 2022, vol. 25, no. 4, pp. 32-38. doi: https://doi.org/10.15407/pmach2022.04.032.
Hontarovskyi P.P., Smetankina N.V., Ugrimov S.V., Garmash N.H., Melezhyk I.I. Computational Studies of the Thermal Stress State of Multilayer Glazing with Electric Heating. Journal of Mechanical Engineering, 2022, vol. 25, no. 1, pp. 14-21. doi: https://doi.org/10.15407/pmach2022.02.014.
Kostikov A.O., Zevin L.I., Krol H.H., Vorontsova A.L. The Optimal Correcting the Power Value of a Nuclear Power Plant Power Unit Reactor in the Event of Equipment Failures. Journal of Mechanical Engineering, 2022, vol. 25, no. 3, pp. 40-45. doi: https://doi.org/10.15407/pmach2022.03.040.
Rusanov A.V., Subotin V.H., Khoryev O.M., Bykov Y.A., Korotaiev P.O., Ahibalov Y.S. Effect of 3D Shape of Pump-Turbine Runner Blade on Flow Characteristics in Turbine Mode. Journal of Mechanical Engineering, 2022, vol. 25, no. 4, pp. 6-14. doi: https://doi.org/10.15407/pmach2022.04.006.
Sushchenko O., Averyanova Y., Ostroumov I., Kuzmenko N., Zaliskyi M., Solomentsev O., Kuznetsov B., Nikitina T., Havrylenko O., Popov A., Volosyuk V., Shmatko O., Ruzhentsev N., Zhyla S., Pavlikov V., Dergachov K., Tserne E. Algorithms for Design of Robust Stabilization Systems. Computational Science and Its Applications – ICCSA 2022. ICCSA 2022. Lecture Notes in Computer Science, 2022, vol. 13375, pp. 198-213. doi: https://doi.org/10.1007/978-3-031-10522-7_15.
Zhyla S., Volosyuk V., Pavlikov V., Ruzhentsev N., Tserne E., Popov A., Shmatko O., Havrylenko O., Kuzmenko N., Dergachov K., Averyanova Y., Sushchenko O., Zaliskyi M., Solomentsev O., Ostroumov I., Kuznetsov B., Nikitina T. Statistical synthesis of aerospace radars structure with optimal spatio-temporal signal processing, extended observation area and high spatial resolution. Radioelectronic and Computer Systems, 2022, no. 1, pp. 178-194. doi: https://doi.org/10.32620/reks.2022.1.14.
Wang D., Yu Q. Review on the development of numerical methods for magnetic field calculation of ships. Ships Science and Technology, 2014, vol. 36, no. 3, pp. 1-6.
Jin H., Wang H., Zhuang Z. A New Simple Method to Design Degaussing Coils Using Magnetic Dipoles. Journal of Marine Science and Engineering, 2022, vol. 10, no. 10, art. no. 1495. doi: https://doi.org/10.3390/jmse10101495.
Chadebec O., Rouve L.-L., Coulomb J.-L. New methods for a fast and easy computation of stray fields created by wound rods. IEEE Transactions on Magnetics, 2002, vol. 38, no. 2, pp. 517-520. doi: https://doi.org/10.1109/20.996136.
Baranov M.I., Rozov V.Y., Sokol Y.I. To the 100th anniversary of the National Academy of Sciences of Ukraine – the cradle of domestic science and technology. Electrical Engineering & Electromechanics, 2018, no. 5, pp. 3-11. doi: https://doi.org/10.20998/2074-272X.2018.5.01.
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2024 B. I. Kuznetsov, T. B. Nikitina, I. V. Bovdui, K. V. Chunikhin, V. V. Kolomiets, B. B. Kobylianskyi

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.
Authors who publish with this journal agree to the following terms:
1. Authors retain copyright and grant the journal right of first publication with the work simultaneously licensed under a Creative Commons Attribution License that allows others to share the work with an acknowledgement of the work's authorship and initial publication in this journal.
2. Authors are able to enter into separate, additional contractual arrangements for the non-exclusive distribution of the journal's published version of the work (e.g., post it to an institutional repository or publish it in a book), with an acknowledgement of its initial publication in this journal.
3. Authors are permitted and encouraged to post their work online (e.g., in institutional repositories or on their website) prior to and during the submission process, as it can lead to productive exchanges, as well as earlier and greater citation of published work.




