Robust adaptive fuzzy type-2 fast terminal sliding mode control of robot manipulators in attendance of actuator faults and payload variation
DOI:
https://doi.org/10.20998/2074-272X.2025.1.05Keywords:
robot manipulator, type-2 fuzzy system, fast terminal sliding mode control, adaptive controlAbstract
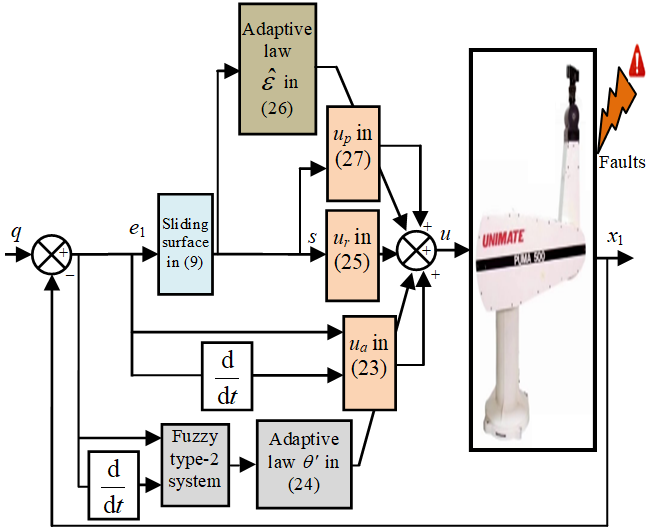
Introduction. This study presents a robust control method for the path following problem of the PUMA560 robot. The technique is based on the Adaptive Fuzzy Type-2 Fast Terminal Sliding Mode Control (AFT2FTSMC) algorithm and is designed to handle actuator faults, uncertainties (such as payload change), and external disturbances. The aim of this study is to utilize the Fast Terminal Sliding Mode Control (FTSMC) approach in order to ensure effective compensation for faults and uncertainties, minimize tracking error, reduce the occurrence of chattering phenomena, and achieve rapid transient response. A novel adaptive fault tolerant Sliding Mode Control (SMC) approach is developed to address the challenges provided by uncertainties and actuator defects in real robotics tasks. Originality. The present work combined the AFT2FTSMC algorithm in order to give robust controllers for trajectory tracking of manipulator’s robot in presence parameters uncertainties, external disturbance, and faults. We use an adaptive fuzzy logic system to estimate the robot’s time-varying, nonlinear, and unfamiliar dynamics. A strong adaptive term is created to counteract actuator defects and approximation errors while also guaranteeing the convergence and stability of the entire robot control system. Novelty. The implemented controller effectively mitigates the chattering problem while maintaining the tracking precision and robustness of the system. The stability analysis has been conducted using the Lyapunov approach. Results. Numerical simulation and capability comparison with other control strategies show the effectiveness of the developed control algorithm. References 53, table 1, figures 8.
References
Capisani L.M., Ferrara A., Magnani L. Second order sliding mode motion control of rigid robot manipulators. 2007 46th IEEE Conference on Decision and Control, 2007, pp. 3691-3696. doi: https://doi.org/10.1109/CDC.2007.4434473.
Wen Yu, Rosen J. Neural PID Control of Robot Manipulators With Application to an Upper Limb Exoskeleton. IEEE Transactions on Cybernetics, 2013, vol. 43, no. 2, pp. 673-684. doi: https://doi.org/10.1109/TSMCB.2012.2214381.
Su Y., Muller P.C., Zheng C. Global asymptotic saturated PID control for robot manipulators. IEEE Transactions on Control Systems Technology, 2010, vol. 18, no. 6, pp. 1280-1288. doi: https://doi.org/10.1109/TCST.2009.2035924.
Tayebi A., Abdul S., Zaremba M.B., Ye Y. Robust Iterative Learning Control Design: Application to a Robot Manipulator. IEEE/ASME Transactions on Mechatronics, 2008, vol. 13, no. 5, pp. 608-613. doi: https://doi.org/10.1109/TMECH.2008.2004627.
Wang M., Yang A. Dynamic Learning From Adaptive Neural Control of Robot Manipulators With Prescribed Performance. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2017, vol. 47, no. 8, pp. 2244-2255. doi: https://doi.org/10.1109/TSMC.2016.2645942.
Duchaine V., Bouchard S., Gosselin C.M. Computationally Efficient Predictive Robot Control. IEEE/ASME Transactions on Mechatronics, 2007, vol. 12, no. 5, pp. 570-578. doi: https://doi.org/10.1109/TMECH.2007.905722.
Feng Lin, Brandt R.D. An optimal control approach to robust control of robot manipulators. IEEE Transactions on Robotics and Automation, 1998, vol. 14, no. 1, pp. 69-77. doi: https://doi.org/10.1109/70.660845.
Morecki A., Knapczyk J. Basics of Robotics: Theory and Components of Manipulators and Robots. Springer, 1999. 580 p.
Vidyasagar M. Robot Dynamics and Control. Wiley, 1989. 336 p.
Jansen H.V., Tas N.R., Berenschot J.W. Encyclopedia of Nanoscience and Nanotechnology. Addison-Wisley, 1989.
Ferrara A., Incremona G.P. Design of an Integral Suboptimal Second-Order Sliding Mode Controller for the Robust Motion Control of Robot Manipulators. IEEE Transactions on Control Systems Technology, 2015, vol. 23, no. 6, pp. 2316-2325. doi: https://doi.org/10.1109/TCST.2015.2420624.
Jin M., Kang S.H., Chang P.H., Lee J. Robust Control of Robot Manipulators Using Inclusive and Enhanced Time Delay Control. IEEE/ASME Transactions on Mechatronics, 2017, vol. 22, no. 5, pp. 2141-2152. doi: https://doi.org/10.1109/TMECH.2017.2718108.
Utkin V. Sliding Modes on Control and Optimization. Springer, 1992. 286 p.
Abed K., Zine H.K.E. Intelligent fuzzy back-stepping observer design based induction motor robust nonlinear sensorless control. Electrical Engineering & Electromechanics, 2024, no. 2, pp. 10-15. doi: https://doi.org/10.20998/2074-272X.2024.2.02.
Xiao B., Hu Q., Zhang Y. Adaptive Sliding Mode Fault Tolerant Attitude Tracking Control for Flexible Spacecraft Under Actuator Saturation. IEEE Transactions on Control Systems Technology, 2012, vol. 20, no. 6, pp. 1605-1612. doi: https://doi.org/10.1109/TCST.2011.2169796.
Liang Y.-W., Xu S.-D., Liaw D.-C., Chen C.-C. A Study of T–S Model-Based SMC Scheme With Application to Robot Control. IEEE Transactions on Industrial Electronics, 2008, vol. 55, no. 11, pp. 3964-3971. doi: https://doi.org/10.1109/TIE.2008.2005138.
Chen G., Song Y., Guan Y. Terminal Sliding Mode-Based Consensus Tracking Control for Networked Uncertain Mechanical Systems on Digraphs. IEEE Transactions on Neural Networks and Learning Systems, 2018, vol. 29, no. 3, pp. 749-756. doi: https://doi.org/10.1109/TNNLS.2016.2636323.
Xu Q. Piezoelectric Nanopositioning Control Using Second-Order Discrete-Time Terminal Sliding-Mode Strategy. IEEE Transactions on Industrial Electronics, 2015, vol. 62, no. 12, pp. 7738-7748. doi: https://doi.org/10.1109/TIE.2015.2449772.
Wang H., Man Z., Kong H., Zhao Y., Yu M., Cao Z., Zheng J., Do M.T. Design and Implementation of Adaptive Terminal Sliding-Mode Control on a Steer-by-Wire Equipped Road Vehicle. IEEE Transactions on Industrial Electronics, 2016, vol. 63, no. 9, pp. 5774-5785. doi: https://doi.org/10.1109/TIE.2016.2573239.
Zheng J., Wang H., Man Z., Jin J., Fu M. Robust Motion Control of a Linear Motor Positioner Using Fast Nonsingular Terminal Sliding Mode. IEEE/ASME Transactions on Mechatronics, 2015, vol. 20, no. 4, pp. 1743-1752. doi: https://doi.org/10.1109/TMECH.2014.2352647.
Madani T., Daachi B., Djouani K. Modular-Controller-Design-Based Fast Terminal Sliding Mode for Articulated Exoskeleton Systems. IEEE Transactions on Control Systems Technology, 2017, vol. 25, no. 3, pp. 1133-1140. doi: https://doi.org/10.1109/TCST.2016.2579603.
Solis C.U., Clempner J.B., Poznyak A.S. Fast Terminal Sliding-Mode Control With an Integral Filter Applied to a Van Der Pol Oscillator. IEEE Transactions on Industrial Electronics, 2017, vol. 64, no. 7, pp. 5622-5628. doi: https://doi.org/10.1109/TIE.2017.2677299.
Van M., Ge S.S., Ren H. Finite Time Fault Tolerant Control for Robot Manipulators Using Time Delay Estimation and Continuous Nonsingular Fast Terminal Sliding Mode Control. IEEE Transactions on Cybernetics, 2017, vol. 47, no. 7, pp. 1681-1693. doi: https://doi.org/10.1109/TCYB.2016.2555307.
Xu S.S.-D., Chen C.-C., Wu Z.-L. Study of Nonsingular Fast Terminal Sliding-Mode Fault-Tolerant Control. IEEE Transactions on Industrial Electronics, 2015, vol. 62, no. 6, pp. 3906-3913. doi: https://doi.org/10.1109/TIE.2015.2399397.
Parra-Vega V., Arimoto S., Yun-Hui Liu, Hirzinger G., Akella P. Dynamic sliding PID control for tracking of robot manipulators: theory and experiments. IEEE Transactions on Robotics and Automation, 2003, vol. 19, no. 6, pp. 967-976. doi: https://doi.org/10.1109/TRA.2003.819600.
Incremona G.P., Ferrara A., Magni L. MPC for Robot Manipulators With Integral Sliding Modes Generation. IEEE/ASME Transactions on Mechatronics, 2017, vol. 22, no. 3, pp. 1299-1307. doi: https://doi.org/10.1109/TMECH.2017.2674701.
Oualah O., Kerdoun D., Boumassata A. Super-twisting sliding mode control for brushless doubly fed reluctance generator based on wind energy conversion system. Electrical Engineering & Electromechanics, 2023, no. 2, pp. 86-92. doi: https://doi.org/10.20998/2074-272X.2023.2.13.
Van M., Ge S.S., Ren H. Robust Fault-Tolerant Control for a Class of Second-Order Nonlinear Systems Using an Adaptive Third-Order Sliding Mode Control. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2016, vol. 47, no. 2, pp. 221-228. doi: https://doi.org/10.1109/TSMC.2016.2557220.
Lilly J.H., Liang Yang. Sliding mode tracking for pneumatic muscle actuators in opposing pair configuration. IEEE Transactions on Control Systems Technology, 2005, vol. 13, no. 4, pp. 550-558. doi: https://doi.org/10.1109/TCST.2005.847333.
Chen J.-Y. Expert SMC-based fuzzy control with genetic algorithms. Journal of the Franklin Institute, 1999, vol. 336, no. 4, pp. 589-610. doi: https://doi.org/10.1016/S0016-0032(97)00077-X.
Tondu B. Artificial Muscles for Humanoid Robots, Humanoid Robots: Human-like Machines, 2007. Book edited by Matthias Hackel, pp. 89-122, Itech, Vienna, Austria.
Braikia K., Chettouh M., Tondu B., Acco P., Hamerlain M. Improved Control Strategy of 2-Sliding Controls Applied to a Flexible Robot Arm. Advanced Robotics, 2011, vol. 25, no. 11-12, pp. 1515-1538. doi: https://doi.org/10.1163/016918611X579510.
Van M., Kang H.-J., Suh Y.-S. Second Order Sliding Mode-Based Output Feedback Tracking Control for Uncertain Robot Manipulators. International Journal of Advanced Robotic Systems, 2013, vol. 10, no. 1, pp. 1-9. doi: https://doi.org/10.5772/55060.
Guezi A., Bendaikha A., Dendouga A. Direct torque control based on second order sliding mode controller for three-level inverter-fed permanent magnet synchronous motor: comparative study. Electrical Engineering & Electromechanics, 2022, no. 5, pp. 10-13. doi: https://doi.org/10.20998/2074-272X.2022.5.02.
Aissaoui M., Bouzeria H., Benidir M., Labed M.A. Harmonics suppression in high-speed railway via single-phase traction converter with an LCL filter using fuzzy logic control strategy. Electrical Engineering & Electromechanics, 2024, no. 2, pp. 16-22. doi: https://doi.org/10.20998/2074-272X.2024.2.03.
Bonivento C., Gentili L., Paoli A. Internal model based fault tolerant control of a robot manipulator. 2004 43rd IEEE Conference on Decision and Control (CDC), 2004, vol. 5, pp. 5260-5265. doi: https://doi.org/10.1109/CDC.2004.1429643.
Bonivento C., Gentili L., Paoli A. Fault Tolerant Tracking of a Robot Manipulator: An Internal Model Based Approach. Systems and Control: Foundations and Applications, 2006, no. 9780817643836, pp. 271-287. doi: https://doi.org/10.1007/0-8176-4470-9_15.
Boukattaya M., Mezghani N., Damak T. Adaptive nonsingular fast terminal sliding-mode control for the tracking problem of uncertain dynamical systems. ISA Transactions, 2018, vol. 77, pp. 1-19. doi: https://doi.org/10.1016/j.isatra.2018.04.007.
Yi S., Zhai J. Adaptive second-order fast nonsingular terminal sliding mode control for robotic manipulators. ISA Transactions, 2019, vol. 90, pp. 41-51. doi: https://doi.org/10.1016/j.isatra.2018.12.046.
Mondal S., Mahanta C. Adaptive second order terminal sliding mode controller for robotic manipulators. Journal of the Franklin Institute, 2014, vol. 351, no. 4, pp. 2356-2377. doi: https://doi.org/10.1016/j.jfranklin.2013.08.027.
Van M., Ge S.S., Ren H. Finite Time Fault Tolerant Control for Robot Manipulators Using Time Delay Estimation and Continuous Nonsingular Fast Terminal Sliding Mode Control. IEEE Transactions on Cybernetics, 2017, vol. 47, no. 7, pp. 1681-1693. doi: https://doi.org/10.1109/TCYB.2016.2555307.
Van M., Do X.P., Mavrovouniotis M. Self-tuning fuzzy PID-nonsingular fast terminal sliding mode control for robust fault tolerant control of robot manipulators. ISA Transactions, 2020, vol. 96, pp. 60-68. doi: https://doi.org/10.1016/j.isatra.2019.06.017.
Xiao B., Yin S., Gao H. Reconfigurable Tolerant Control of Uncertain Mechanical Systems With Actuator Faults: A Sliding Mode Observer-Based Approach. IEEE Transactions on Control Systems Technology, 2018, vol. 26, no. 4, pp. 1249-1258. doi: https://doi.org/10.1109/TCST.2017.2707333.
Amer A.F., Sallam E.A., Elawady W.M. Adaptive fuzzy sliding mode control using supervisory fuzzy control for 3 DOF planar robot manipulators. Applied Soft Computing, 2011, vol. 11, no. 8, pp. 4943-4953. doi: https://doi.org/10.1016/j.asoc.2011.06.005.
Guendouzi A., Boubakir A., Hamerlain M. Higher order sliding mode control of robot manipulator. The Ninth International Conference on Autonomic and Autonomous Systems (ICAS 2013), 2013, pp. 61-66.
Xinghuo Yu, Man Zhihong. Fast terminal sliding-mode control design for nonlinear dynamical systems. IEEE Transactions on Circuits and Systems I: Fundamental Theory and Applications, 2002, vol. 49, no. 2, pp. 261-264. doi: https://doi.org/10.1109/81.983876.
Chen M., Wu Q.-X., Cui R.-X. Terminal sliding mode tracking control for a class of SISO uncertain nonlinear systems. ISA Transactions, 2013, vol. 52, no. 2, pp. 198-206. doi: https://doi.org/10.1016/j.isatra.2012.09.009.
Xiong J.-J., Zhang G.-B. Global fast dynamic terminal sliding mode control for a quadrotor UAV. ISA Transactions, 2017, vol. 66, pp. 233-240. doi: https://doi.org/10.1016/j.isatra.2016.09.019.
Yacef F., Bouhali O., Hamerlain M., Rizoug N. Observer-based Adaptive Fuzzy Backstepping Tracking Control of Quadrotor Unmanned Aerial Vehicle Powered by Li-ion Battery. Journal of Intelligent & Robotic Systems, 2016, vol. 84, no. 1-4, pp. 179-197. doi: https://doi.org/10.1007/s10846-016-0345-0.
Bounar N., Boulkroune A., Boudjema F., M’Saad, M., Farza M. Adaptive fuzzy vector control for a doubly-fed induction motor. Neurocomputing, 2015, vol. 151, part 2, pp. 756-769. doi: https://doi.org/10.1016/j.neucom.2014.10.026.
Mobayen S., Tchier F., Ragoub L. Design of an adaptive tracker for n -link rigid robotic manipulators based on super-twisting global nonlinear sliding mode control. International Journal of Systems Science, 2017, vol. 48, no. 9, pp. 1990-2002. doi: https://doi.org/10.1080/00207721.2017.1299812.
Ma Z., Sun G. Dual terminal sliding mode control design for rigid robotic manipulator. Journal of the Franklin Institute, 2018, vol. 355, no. 18, pp. 9127-9149. doi: https://doi.org/10.1016/j.jfranklin.2017.01.034.
Li T.-H.S., Huang Y.-C. MIMO adaptive fuzzy terminal sliding-mode controller for robotic manipulators. Information Sciences, 2010, vol. 180, no. 23, pp. 4641-4660. doi: https://doi.org/10.1016/j.ins.2010.08.009.
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2025 H. Rahali, S. Zeghlache, B. D. E. Cherif, L. Benyettou, A. Djerioui

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.
Authors who publish with this journal agree to the following terms:
1. Authors retain copyright and grant the journal right of first publication with the work simultaneously licensed under a Creative Commons Attribution License that allows others to share the work with an acknowledgement of the work's authorship and initial publication in this journal.
2. Authors are able to enter into separate, additional contractual arrangements for the non-exclusive distribution of the journal's published version of the work (e.g., post it to an institutional repository or publish it in a book), with an acknowledgement of its initial publication in this journal.
3. Authors are permitted and encouraged to post their work online (e.g., in institutional repositories or on their website) prior to and during the submission process, as it can lead to productive exchanges, as well as earlier and greater citation of published work.




