Tilt-fractional order proportional integral derivative control for DC motor using particle swarm optimization
DOI:
https://doi.org/10.20998/2074-272X.2023.2.03Keywords:
DC motor, speed control, fractional order proportional integral derivative, particle swarm optimizationAbstract
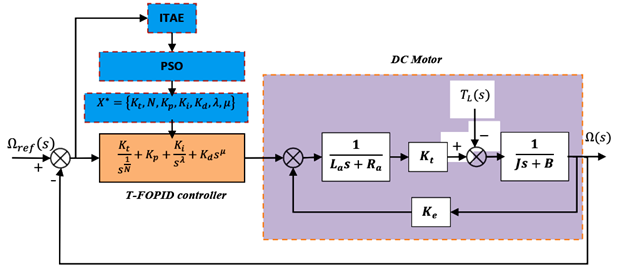
Introduction. Recently, the most desired goal in DC motor control is to achieve a good robustness and tracking dynamic of the set-point reference speed of the feedback control system. Problem. The used model should be as general as possible and consistently represent systems heterogeneous (which may contain electrical, mechanical, thermal, magnetic and so on). Goal. In this paper, the robust tilt-fractional order proportional integral derivative control is proposed. The objective is to optimize the controller parameters from solving the criterion integral time absolute error by particle swarm optimization. The control strategy is applied on DC motor to validate the efficiency of the proposed idea. Methods. The proposed control technique is applied on DC motor where its dynamic behavior is modeled by external disturbances and measurement noises. Novelty. The proposed control strategy, the synthesized robust tilt-fractional order proportional integral derivative speed controller is applied on the DC motor. Their performance and robustness are compared to those provided by a proportional integral derivative and fractional order proportional integral derivative controllers. Results. This comparison reveals superiority of the proposed robust tilt-fractional order proportional integral derivative speed controller over the remaining controllers in terms of robustness and tracking dynamic of the set-point reference speed with reduced control energy.
References
Podlubny I. Fractional-order systems and PID-controllers. IEEE Transactions on Automatic Control, 1999, vol. 44, no. 1, pp. 208-214. doi: https://doi.org/10.1109/9.739144.
Monje C.A., Vinagre B.M., Feliu V., Chen Y. Tuning and auto-tuning of fractional order controllers for industry applications. Control Engineering Practice, 2008, vol. 16, no. 7, pp. 798-812. doi: https://doi.org/10.1016/j.conengprac.2007.08.006.
Amieur T., Sedraoui M., Amieur O. Design of Robust Fractional-Order PID Controller for DC Motor Using the Adjustable Performance Weights in the Weighted-Mixed Sensitivity Problem. IAES International Journal of Robotics and Automation (IJRA), 2018, vol. 7, no. 2, pp. 108-118. doi: https://doi.org/10.11591/ijra.v7i2.pp108-118.
Regad M., Helaimi M., Taleb R., Gabbar H., Othman A. Optimal frequency control in microgrid system using fractional order PID controller using krill herd algorithm. Electrical Engineering & Electromechanics, 2020, no. 2, pp. 68-74. doi: https://doi.org/10.20998/2074-272X.2020.2.11.
Barbosa R.S., Tenreiro Machado J.A., Jesus I.S. Effect of fractional orders in the velocity control of a servo system. Computers & Mathematics with Applications, 2010, vol. 59, no. 5, pp. 1679-1686. doi: https://doi.org/10.1016/j.camwa.2009.08.009.
Tavakoli-Kakhki M., Haeri M. Fractional order model reduction approach based on retention of the dominant dynamics: Application in IMC based tuning of FOPI and FOPID controllers. ISA Transactions, 2011, vol. 50, no. 3, pp. 432-442. doi: https://doi.org/10.1016/j.isatra.2011.02.002.
Ardjal A., Mansouri R., Bettayeb M. Fractional sliding mode control of wind turbine for maximum power point tracking. Transactions of the Institute of Measurement and Control, 2019, vol. 41, no. 2, pp. 447-457. doi: https://doi.org/10.1177/0142331218764569.
Li M., Zhou P., Zhao Z., Zhang J. Two-degree-of-freedom fractional order-PID controllers design for fractional order processes with dead-time. ISA Transactions, 2016, vol. 61, pp. 147-154. doi: https://doi.org/10.1016/j.isatra.2015.12.007.
Chen K., Tang R., Li C. Phase-constrained fractional order PIλ controller for second-order-plus dead time systems. Transactions of the Institute of Measurement and Control, 2017, vol. 39, no. 8, pp. 1225-1235. doi: https://doi.org/10.1177/0142331216634427.
Aidoud M., Sedraoui M., Lachouri A., Boualleg A. A robustification of the two degree-of-freedom controller based upon multivariable generalized predictive control law and robust H∞ control for a doubly-fed induction generator. Transactions of the Institute of Measurement and Control, 2018, vol. 40, no. 3, pp. 1005-1017. doi: https://doi.org/10.1177/0142331216673425.
Patra S., Sen S., Ray G. A linear matrix inequality approach to parametric H∞ loop shaping control. Journal of the Franklin Institute, 2011, vol. 348, no. 8, pp. 1832-1846. doi: https://doi.org/10.1016/j.jfranklin.2011.05.006.
Amieur T., Bechouat M., Sedraoui M., Kahla S., Guessoum H. A new robust tilt-PID controller based upon an automatic selection of adjustable fractional weights for permanent magnet synchronous motor drive control. Electrical Engineering, 2021, vol. 103, no. 3, pp. 1881-1898. doi: https://doi.org/10.1007/s00202-020-01192-3.
Aashoor F.A.O., Robinson F.V.P. Maximum power point tracking of PV water pumping system using artificial neural based control. 3rd Renewable Power Generation Conference (RPG 2014), Naples, 2014, pp. 1-6, doi: https://doi.org/10.1049/cp.2014.0923.
Aghababa M.P. Optimal design of fractional-order PID controller for five bar linkage robot using a new particle swarm optimization algorithm. Soft Computing, 2016, vol. 20, no. 10, pp. 4055-4067. doi: https://doi.org/10.1007/s00500-015-1741-2.
Hekimoglu B. Optimal Tuning of Fractional Order PID Controller for DC Motor Speed Control via Chaotic Atom Search Optimization Algorithm. IEEE Access, 2019, vol. 7, pp. 38100-38114. doi: https://doi.org/10.1109/ACCESS.2019.2905961.
Anwar N., Hanif A., Ali M.U., Zafar A. Chaotic-based particle swarm optimization algorithm for optimal PID tuning in automatic voltage regulator systems. Electrical Engineering & Electromechanics, 2021, no. 1, pp. 50-59. doi: https://doi.org/10.20998/2074-272X.2021.1.08.
Bouraghda S., Sebaa K., Bechouat M., Sedraoui M. An improved sliding mode control for reduction of harmonic currents in grid system connected with a wind turbine equipped by a doubly-fed induction generator. Electrical Engineering & Electromechanics, 2022, no. 2, pp. 47-55. doi: https://doi.org/10.20998/2074-272X.2022.2.08.
Marini F., Walczak B. Particle swarm optimization (PSO). A tutorial. Chemometrics and Intelligent Laboratory Systems, 2015, vol. 149, pp. 153-165. doi: https://doi.org/10.1016/j.chemolab.2015.08.020.
Kao C.-C., Chuang C.-W., Fung R.-F. The self-tuning PID control in a slider–crank mechanism system by applying particle swarm optimization approach. Mechatronics, 2006, vol. 16, no. 8, pp. 513-522. doi: https://doi.org/10.1016/j.mechatronics.2006.03.007.
Kumar Sahu R., Panda S., Biswal A., Chandra Sekhar G.T. Design and analysis of tilt integral derivative controller with filter for load frequency control of multi-area interconnected power systems. ISA Transactions, 2016, vol. 61, pp. 251-264. doi: https://doi.org/10.1016/j.isatra.2015.12.001.
Barisal A.K. Comparative performance analysis of teaching learning based optimization for automatic load frequency control of multi-source power systems. International Journal of Electrical Power & Energy Systems, 2015, vol. 66, pp. 67-77. doi: https://doi.org/10.1016/j.ijepes.2014.10.019.
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2022 T. Amieur, D. Taibi, S. Kahla, M. Bechouat, M. Sedraoui

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.
Authors who publish with this journal agree to the following terms:
1. Authors retain copyright and grant the journal right of first publication with the work simultaneously licensed under a Creative Commons Attribution License that allows others to share the work with an acknowledgement of the work's authorship and initial publication in this journal.
2. Authors are able to enter into separate, additional contractual arrangements for the non-exclusive distribution of the journal's published version of the work (e.g., post it to an institutional repository or publish it in a book), with an acknowledgement of its initial publication in this journal.
3. Authors are permitted and encouraged to post their work online (e.g., in institutional repositories or on their website) prior to and during the submission process, as it can lead to productive exchanges, as well as earlier and greater citation of published work.




